Unit Labor Cost (ULC) is figured by taking Total Labor Cost (TLC) and dividing it by real output (RGDP):
Work backwards and multiply both sides by real output to get Total Labor Cost:
I put that number on a FRED graph along with the Monetary Interest Paid number from yesterday:
 |
| Graph #1: Oops! |
Ouch. Labor cost dwarfs interest cost.
Can that be right??
No, I messed up. Read the units in the left-hand border. It's an "index" (the Unit Labor Cost number is an index) times billions of chained 2009 dollars, for the blue line. But the 2009 value of the index is 100. So Graph #1, for 2009, shows a number that is 100 times what it should be. For all the years, 100 times what it should be.
I have to take the ULCNFB*GDPC1 formula (in the top border of Graph #1) and divide it by 100:
.png) |
| Graph #2: Total Labor Cost (blue) and Monetary Interest Paid by Domestic Business (red) |
Still, the cost of labor is far more than the cost of interest.
Fair enough. And I should hope so! Nonetheless, I argue that it is the increasing cost of interest that is the source of our economic troubles, going back to the 1960s or before.
High numbers on a graph like this squeeze the low numbers down near zero and you lose the detail. You can't see what's happening in the early years. So, to compare the two lines, divide one by the other:
 |
| Graph #3: Total Labor Cost relative to Monetary Interest Paid by Domestic Business |
Graph #3 look familiar? Looks just like yesterday's Graph #4, Unit Labor Cost relative to Unit Monetary Interest Cost.
Well, yeah. It's only a slight re-arrangement of the same source numbers.
Graph #3 here is better though. The numbers on the vertical scale are better. The high point of the blue line here is between 70 and 80. On yesterday's graph it was between 7000 and 8000. Yesterday, I didn't even know what the numbers meant.
The reason these numbers are lower? Because I divided by 100 to fix my error. 7000 divided by 100 is 70, and 8000 divided by 100 is 80, so that's how the numbers changed. And suddenly the numbers become meaningful.
Back before the 1950s, total labor cost was about 70 times the total cost of interest paid by domestic US businesses. From 1980 to the crisis it was generally less than 10 times as much. That means that for every $70 US business paid to labor in the late 1940s, they had one dollar of interest cost. Since 1980, for every $70 US business paid to labor, they've had about $7 to $10 of interest cost, maybe more. That's what the graph says.
Here, let me invert the ratio:
 |
| Graph #4: Monetary Interest Paid by Domestic Business, relative to Total Labor Cost |
If we had kept the interest cost to US business at four cents per dollar of labor cost, there would have been a whole lot of money left over to boost business profits. The economy would have grown like gangbusters.
Some of those boosted profits could have been spent giving people raises. That would have increased aggregate demand and living standards... and would likely have quelled the anger that vents itself these days in politics.
If interest costs were restrained, the financial sector would not have grown so much. Finance would today be a smaller segment of GDP. But then, with higher profits in the nonfinancial sector, GDP would have grown much more.
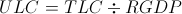


3 comments:
Hi Art,
There is something not right with ULCNFP*GDPC1/100. It suggests that total labor costs are greater than GDP before 2009.
http://research.stlouisfed.org/fred2/graph/?g=r7t
Well that's significant. I don't know, Jim. I recreated your r7t graph for myself so I could look at the parts, and ended up with just the same result you got.
Trying to imagine what could be wrong. Something in my assumptions, I assume...
Perhaps, certainly, ULCNFB does not include all of labor: "Nonfarm Business Sector: Unit Labor Cost" excludes farm labor, at least.
Okay, but say ULCNFB covers 80% of labor. Then the "total labor cost" we get after the calculation should be 80% of labor cost... should be LESS than GDP, not more. In addition, it should be less because not all income is labor income, so it might drop to 60% of GDP or something.
So that doesn't fix the problem.
If the calc give a result that is too big, then either the ULC number is too big or the GDPC1 number is too big.
Maybe that's it. Maybe it's not right to use GDPC1 for output, because the output of labor is some percentage of GDPC1 that is less than 100%.
That's probably it. But, how to fix it? Any ideas?
I don't think your calculations are the problem. I think its "Unit Labor Cost" is a bit of a bogus concept that doesn't tell you much. Its kind of the national ratio of apples to oranges.
Post a Comment